Published:
13.04.2016
Road traffic, in addition to the movement itself implied in the name, involves stopping. You brake at a traffic light, before a pedestrian crossing, in front of another car, and the like many times during a trip. Sometimes (usually more often than you would like) you have to stop very quickly and abruptly. There are 2 terms that need to be distinguished in this regard: braking distance and stopping distance. They mean different things, although they are interconnected by a common purpose: to show the driver that the laws of inertia apply to any car.
Economics 9th grade
The window regulator failed 7 reasons and solutions
“Mining and chemical industry” - Industrial raw materials. Cphene CaTiO (SiO4). Noble metals. 2. Non-metallic minerals. Platinum. Mining chemical raw materials. FERSMAN ALEXANDER EVGENIEVICH August 30, 1921. 1. Metallic minerals. Black metals. Pentlandite (Fe,Ni)9S8. Pyrite FeS2. Production of phosphate fertilizers. Eudialyte Na4Ca2Zr(Si3O9). A.S. Pushkin. Oh, how many wonderful discoveries the spirit of enlightenment is preparing for us! Gold.
“Branches of food and light industry” - Koryazhma. Murmansk and Astrakhan. 5. Industries for the primary processing of flax are located taking into account the factor: 9. Which city does not have a flax industry: Both are incorrect. Bakery - yes, sugar - no. Lipetsk and Tambov regions. Nakhodka and Omsk. Voronezh and Belgorod regions. Consumer. Consumer. Shoe. Electric power. Labor. Transport. Ecological. Both are correct.
“Market infrastructure” - Place of birth. Job search. Capital market. Unemployment. Summary. Demand. Consumer market. Market infrastructure. Factors of production market. Market. Labor market. System of social mechanisms.
"Metallurgy of Russia" - Non-ferrous metallurgy. Imported concentrates are used. To study the features of the Russian metallurgical complex. Lead-zinc industry. Metallurgical bases. Ore Energy Consumer. The oldest branch of non-ferrous metallurgy in our country. The metallurgical complex includes ferrous and non-ferrous metallurgy. Placement factors. Find the definition of a metallurgical complex. Data. Aluminum industry.
“Russia in the global economy” - Debtors use external loans. Centers of the world economy: Exploitation of natural resources. It has passed the stage of industrialization. Ecological deterioration. The world is in the post-industrial stage. Resource-saving technologies. Characteristic features of highly developed countries: Periphery - the poorest countries: Paths of development of Russia. Show “+” and “–” of the post-industrial stage of development of society. + The driving force is knowledge, intelligence.
“The problem of unemployment” - Causes of unemployment. Registration of citizens as individual entrepreneurs. The reasons that make the problem of unemployment acute. Methods of combating unemployment. Types of unemployment. K. Charles (American inventor). Directions for reducing unemployment at this stage in the Republic of Tatarstan. Has a variety of skills. How to avoid becoming unemployed or survive unemployment? Dynamics of the number of registered unemployed.
"Economics 9th grade"
so UNT / Traffic rules / Lesson plans for traffic rules 6th grade
Lesson No. 3 Topic: “Braking and stopping distance of vehicles”
26.09.2013 29719 0
Goals and objectives of the lesson:
creating a sense of danger when crossing the roadway in front of nearby vehicles; familiarizing students with the concepts of braking and stopping distance, danger zone.
Materials for the lesson:
Let's imagine that we all have a license to drive a car, that we are drivers. We get behind the wheel, turn on the ignition, start the engine. Go. We're driving down the street. The weather is clear, the asphalt is dry, the mood is excellent. We are not daredevil racers, but ordinary experienced drivers.
The speedometer needle, shaking, shows: 20, 30, 40. We are driving at a speed of 40 km/h - this is a sufficient and quite moderate speed for driving around the city in a passenger car. You can’t say anything: it’s nice to sit behind the wheel and drive the car. We carefully monitor the road, and also look at the sidewalk - from there an inattentive pedestrian can unexpectedly jump out onto the pavement. And suddenly a boy runs out onto the road, right in front of our car. We press the brake pedal... From the moment the driver notices the danger until the moment he presses the brake pedal, some time passes, which is called the driver's reaction time
(RA).
This time is very short and may seem insignificant, but it is of great importance. In a healthy person it is 0.8-1.0 seconds. While the human brain “telegraphs”—instructs the leg to stop—the car moves on. This is the distance traveled
by the car
during the driver's reaction time
(RT).
From the moment you press the brake pedal, the wheels begin to rotate more slowly, even stopping, but the car has not stopped yet. The path a car travels from the start of braking to a complete stop is called the braking path
or
braking distance
(PT).
stopping distance
And the path that a car travels from the moment the driver notices the danger until it comes to a complete stop is called stopping distance
(PTL).
Thus, stopping distance (PTL) = distance covered during the driver's reaction time (RT) + braking distance (PT).
РТL = RT + РТ
Fortunately, the boy escaped with fright; he turned pale and was ready to cry. It's good that it is so. One of the eyewitnesses managed to call the ambulance station (phone 03) and the police department (phone 02), and soon an ambulance rushed with the siren on, followed by a traffic inspector. The doctor examined the boy and found no injuries or even scratches.
The traffic inspector wrote down the boy's name, school number, home address and sent him home.
But everything could have ended differently. How? (Students list all the possible consequences of the boy’s action).
The boy could have been injured and could have remained disabled. To avoid hitting the boy, the driver could turn right or left, being unable to stop the car, drive onto the sidewalk and hit pedestrians. The driver could veer off the road, hit a pole or building, get injured, etc.
But the work of the traffic inspector at the scene did not end there. Or rather, it has just begun. It was necessary to establish how fast the car was traveling and whether the driver adhered to the traffic rules. First, the traffic inspector measured the brake mark.
When investigating the causes of a traffic accident, this is the most important thing, because the braking distance can be used to determine the speed of the car.
The length of the braking mark was 12 meters. This is the stopping distance
, the distance the car has traveled from the start of braking to a complete stop. Then the traffic inspector took out a table from the folder, calculated it and told the driver that he was driving at a speed of 40 km/h. And he noticed the boy on the road 20-25 meters away. How did he know this? We'll do the math too.
Let us remember that the braking distance (PT) + the distance covered during the driver's reaction time (RT) = stopping distance (PTL). We already know the length of the braking distance; it is 12 meters. You need to find out the length of the path covered during the driver's reaction time. Let's assume that the driver's reaction time is 1 second. How many meters will a car travel in one second if its speed is 40 km/h?
1 hour is 60 minutes, 1 minute is 60 seconds, which means 1 hour = 3600 seconds.
1 kilometer = 1000 meters, 40 kilometers = 40,000 meters.
40000 :
3600 = 11.1 meters. This is the distance covered during the driver's reaction time (RT).
And the stopping distance length (PTL):
12 m + 11.1 m = 23.1 m.
At a distance of 23.1 meters, the driver saw a boy running. If he had noticed it at a shorter distance, the consequences would have been sad, misfortune would have become inevitable. When driving on a flat, dry asphalt road at a speed of 40 km/h, the driver of a passenger car is able to stop the car 23 meters after he notices the danger. This distance is called the danger zone
, no one can get here.
Each moving vehicle has its own danger zone
in front of the car, the length of this zone depends on:
— vehicle speed;
— from the weight of the car;
— on the track profile (evenness, presence of ascents and descents);
- on the condition of the coating and the quality of the tread on the tires.
Driving speed and danger zone
The higher the speed of the car, the longer the distance it travels in one second. As speed increases, the first thing that increases is the distance the car travels during the driver’s reaction time. The higher the speed, the greater the force developed in the wheels, the longer the braking distance and the danger zone.
Length of the danger zone in meters on a dry asphalt road at various car speeds:
| Speed (km/h) | Distance traveled in 1 second of driver reaction time (RT) (m) | Braking distance (RT) (m) | Danger zone (PTL) (RT + RT) (m) |
| 10 | 2,8 | 0,8 | 3,6 |
| 30 | 8,3 | 7,2 | 15,5 |
| 50 | 13,9 | 19,6 | 33,5 |
| 60 | 16,7 | 28,4 | 45,1 |
| 80 | 22,2 | 43,2 | 65,4 |
| 100 | 27,8 | 78,7 | 106,5 |
| 120 | 33,3 | 113,0 | 146,3 |
If the speed doubles, the danger zone increases at the same time three times.
This means that crossing the road in front of an approaching car is dangerous.
You should never think: “Nothing, I’ll have time...” and then start crossing the road. If, for example, a passenger car is approaching at a speed of 60 km/h, then crossing the street 50 meters from it is already risky; you may find yourself on the border of a danger zone. It is safe to cross the road only when there is a distance equal to the length of two danger zones to the approaching car.
Naturally, it is difficult for a pedestrian to accurately determine the speed of an approaching car and calculate the length of the danger zone. No pedestrian is capable of this, so you should “err” in your favor when estimating the distance to the approaching car. This is the best way and a guarantee of safety.
It is especially difficult to determine the distance of an approaching car and its approximate speed at dusk, in the dark, in fog, in rain and snowfall. At dusk and in the dark, visibility deteriorates significantly. Pedestrians and drivers are not very good at distinguishing what is happening on the road. In the dark, it is easy to make a mistake in determining the distance both to a moving car and to stationary objects. In poor lighting, close objects often appear far away, and distant objects appear close. Visual illusions occur: a stationary object can be mistaken for moving, a moving object for an approaching one, and vice versa. In the dark, it is difficult to determine by the light of a car's headlights whether it is approaching or not. And if it is approaching, then at what speed?
Human eyes are designed in such a way that they need time to get used to the dark. But still, night vision is significantly worse than day vision.
In poor lighting, at dusk, the eyes have difficulty distinguishing colors. For example, red appears dark and even black. Green appears lighter than red. When approaching a traffic light, its signals initially appear white, and only later do we begin to distinguish colors. First of all, green becomes visible, then yellow and red. Unfortunately, not all pedestrians and drivers know this and do not exercise due caution in the dark.
The conclusion is simple. In the dark, when crossing the street, the distance to an approaching car should be greater than during the day. You can’t rush during the day, and even more so in the dark. It is advisable not to wear dark clothes in the dark. If possible, you should use reflective stickers, clothing tapes and badges that help drivers see you from a long distance.
This is how much a driver needs to know and take into account when choosing the speed of his car, and a pedestrian - when determining the distance to an approaching car if he needs to cross the road.
When the driver chooses the driving speed, he knows the technical condition of his car and his capabilities. Therefore, he also knows the length of the car’s braking distance.
The pedestrian does not know the technical condition of the approaching car, nor what physical condition the driver is in, what his skills and experience are.
This means that for a pedestrian, when crossing the road, it is very important to choose the right distance to an approaching car or other vehicle. You cannot cross the road in places where the driver does not expect a pedestrian to appear on the roadway, i.e. where there is no pedestrian crossing. A pedestrian must know where and how to cross the road, and follow these rules very precisely.
Vehicle weight and danger zone
The greater the mass of the vehicle, the greater its energy. Therefore, it turns out that at the same speed, different vehicles have different braking distances, and, consequently, a dangerous zone. In a truck, the weight of the load plays a role, because a vehicle without a load is easier to stop than a loaded one. The load increases the stopping distance of a truck by approximately one third
Road surface condition and danger zone
The condition of the pavement has the greatest influence on the length of the braking distance. The rougher the road surface, the better the grip of the wheels on the road. When the wheels begin to rotate more slowly when braking, there is no danger of slipping. For braking, this circumstance is of great importance. Our roads are covered with asphalt concrete, asphalt, crushed stone and gravel. They have different degrees of roughness. Drivers like the most asphalt covered with crushed stone. Smooth asphalt is much worse. Asphalt covered with crushed stone can be compared to sandpaper, and smooth asphalt can be compared to ordinary paper, say, a sheet from a notebook. Try running your finger over the sandpaper. Somehow the grip of the fingers with the paper occurs on its own. It's a different matter with smooth paper. Fingers slide on the paper, grip is much worse. The situation is exactly the same with the grip of tires and the road on different surfaces.
Meteorological conditions have a particularly great influence on the condition of the coating. Tires have the best grip on the road when the surface is dry. Grip greatly deteriorates when the surface is wet.
The onset of rain is especially deceptive and poses a great danger to drivers and pedestrians. The first drops of rain that fall on the road mix with dust and cover the road with a slimy layer. This significantly worsens the traction of the tires to the road, driving the car is difficult, the car can skid when turning, and a sharp turn of the steering wheel can cause a side slip.
When it starts to rain, motorcyclists find themselves in the most dangerous situation. The two-wheeled motorcycle becomes difficult to control, the wheels begin to slip, and the motorcycle may tip over. After some time, the rain will wash away the mucous layer from the road, and the tires’ grip on the road will improve slightly, but will still not be as good as before. Why?
The thing is that when a car wheel rotates, it cannot completely displace the water that has entered the tread grooves. A layer of water remains between the tire and the road surface; the car rides as if on a cushion of water and is therefore unstable. When driving fast, the car seems to float on the road. This phenomenon is called aquasliding. It is essentially similar to water skiing. The incredible power of a raindrop on the road. It will make even the heaviest truck slide. The more worn the tires are, the more likely they are to skid.
A road with compacted snow and especially ice is several times more dangerous than a wet road. The braking distance here increases especially sharply.
Rain, snow and ice are unwelcome companions for the driver. The braking distance, as well as the danger zone, increases by two times on a packed snow road, and by five times on ice. Stopping a car in winter is much more difficult than in summer.
Careless pedestrians cause a lot of trouble for drivers. It seems to them that it is just as easy to stop a car in winter as in summer. We have already explained that this is not so. The danger zone in front of the car is now increased several times. And an inattentive pedestrian, who doesn’t care about all this, crosses the road in front of a car, which, as it seems to him, is still quite far away. The driver instinctively presses the brake pedal. But since the adhesion of the wheels to the road is poor, the wheels stop rotating, become blocked, and the car slides further like a sled, not obeying the steering wheel control.
Every pedestrian must understand that any of his actions, no matter how insignificant, as it seems to him, can cause a dangerous situation. It’s easy to prevent this - let’s all be truly attentive and polite! And in traffic the word “please” is applicable. Let's put it into practice!
Review questions
1. Why is it impossible to stop a car instantly?
2. What is stopping distance?
3. What is the distance traveled during the driver’s reaction time?
4. What is a danger zone?
5. One car is traveling at a speed of 10 km/h, and the other is traveling at 30 km/h. Which one will stop first if the drivers brake at the same time?
6. Why shouldn’t you cross the road in front of an approaching car?
7. When and in what case is it easier to stop a car: in summer or winter, on a dry or wet road?
8. Do you think that a pedestrian and a cyclist also have a braking distance?
9. If a truck and a car are traveling at the same speed, which one will stop first after braking?
10. How many times does the danger zone increase in winter compared to a dry road in summer?
11. From Tallinn to Narva 216 kilometers. How many hours will it take a motorcyclist to cover this distance if he travels at a speed of 60 km/h (one kilometer per minute)?
12. Why is driving at high speed dangerous?
Theoretical approach
Rules for turning around tram tracks outside an intersection
The braking distance of any car is usually called the distance that the vehicle covers in the period of time from pressing the brake pedal to the final stop. This parameter is influenced by the following factors:
- Movement speed;
- Type of road surface;
- Wear of the car brake system;
- Tire brand and condition.
To calculate the stopping distance, there is a formula: S = Ke * V * V/(254 * Fc),
where S is the braking distance,
Ke – value of the braking coefficient (for passenger cars Ke=1),
V – speed at the moment of braking (km/h),
Фc – the value of the coefficient characterizing adhesion to the road surface.
Phosphorus levels are strongly influenced by atmospheric factors. Example: Fs of dry asphalt = 0.7; wet asphalt – 0.4; compacted snow - 0.2, and ice - 0.1.
When a driver is driving along a highway, he cannot mentally calculate his braking distance, so averages apply for these cases. These figures are indicative only and are calculated for routes with normal conditions. For example, at a speed of 60 km/h the braking distance is 17 m long, at 90 km/h – from 50 to 60 m, at 90 km/h – 90 m and above.
Important: exceeding the speed by 2 times results in a 4-fold increase in braking distance!
Practical advice
But other factors also influence the length of a car's braking distance. When an obstacle on the road comes into the driver's field of vision, he should intuitively press the brake pedal. Professional racers perform this operation in 0.3 seconds, while a beginner spends 1.7 seconds or more on the manipulation.
The brake system also takes time to operate. In passenger cars, the duration of brake actuation is from 0.1 to 0.3 seconds, the next 0.3-0.4 seconds the braking force increases to the limit. These are, of course, fractions of seconds, but they are the ones that bring the car body closer to the obstacle.
To reduce the negative effects of sudden braking, the driver must follow the following rules:
1) Realistically assess the condition of the road surface and compare the speed developed with it;
2) Sufficient distance from the car in front;
3) Newer cars have a shorter braking distance;
4) You cannot change lanes in front of oncoming buses and trucks, whose braking distance is much longer;
5) While driving, you should not be distracted from the road;
6) Regular inspection of brake hoses and pads will allow you to timely identify the malfunction and eliminate it.
You should not press the brake pedal sharply; on the road you need to remain calm and pay close attention to other road users. The described measures will allow you to avoid many emergency situations.
And then what
It is possible to calculate the braking distance of a car, but we believe that this is pointless. It’s somehow hard to imagine that a driver, especially an inexperienced one, at the moment when he needs to make a decision, begins to substitute numbers into the formula. There are averages, and you should focus on them. Even if they are approximate, they can tell you the order of the numbers, the “fork” of values. There are tables of car braking distance lengths depending on its class, because, of course, a heavy SUV brakes completely differently than a minicar. These tables are compiled for a speed of 60 km/h. But be careful, the proportion for the calculation is insidious, it multiplies the values by the square of the “delta”, that is, when the speed increases by 2 times, the braking distance will increase by 4 times!
Braking with ABS
Example of vehicle calculation
The ABS system works precisely on the principle of stepped braking, and its main task is to prevent the car from going into an uncontrolled skid. ABS does not completely block the wheels, thereby leaving the driver in control of the vehicle's movement. Extensive testing has shown that ABS will shorten stopping distances on dry or wet pavement, and also works great on gravel. But in other conditions the system partially loses its value.
In winter conditions, ABS will increase the braking distance by 15-30 meters when driving on snow or ice
At the same time, the system will leave the driver control over the car, which can be critically important when driving on ice.
Friction table at different speeds
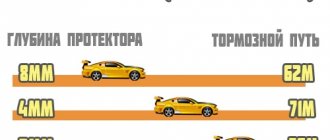
What is the braking and stopping distance of a car?
Road traffic, in addition to the movement itself implied in the name, involves stopping. You brake at a traffic light, before a pedestrian crossing, in front of another car, and the like many times during a trip. Sometimes (usually more often than you would like) you have to stop very quickly and abruptly. There are 2 terms that need to be distinguished in this regard: braking distance and stopping distance. They mean different things, although they are interconnected by a common purpose: to show the driver that the laws of inertia apply to any car.
What factors influence braking and braking distance?
Of course, what matters is the speed of the car at which it moves along the road. The braking distance is also affected by the quality of the brake system installed on the car. The condition of the road undoubtedly plays an important role (snow, ice, quality of asphalt/concrete, cracks in the road surface, leaves, puddles, etc.). And of course, don’t forget about the condition of your car’s tires. Indeed, in certain cases, worn-out tires will greatly increase the car’s braking distance, since they will not be able to transfer normal braking power to the road surface, unlike new tires that have normal grip.
It is also clear that on a wet surface the braking distance of a car is greater than on dry asphalt.
Don’t forget about the driver’s level of training. Particularly important, as we have learned, for the final braking distance is the speed of the driver’s reaction to a traffic situation that requires stopping the car. But the speed of reaction behind the wheel depends not only on driving experience. For example, did you know that when you get behind the wheel in a sleepy state (didn't get enough sleep, are tired, or have been driving for a long time), your reaction speed can slow down by almost half compared to the reaction speed of a well-rested driver.
In general, the speed of decision-making while driving (reaction speed) is influenced by many factors: the age of the driver, alcohol or hangover, the use of certain medications and overall health status. Thus, with many chronic diseases, the reaction speed of many drivers is significantly reduced. Consequently, all these factors seriously affect the braking distance of the car.
Which car has a longer braking distance - a loaded one or an empty one? More than half of people will answer that it is loaded. How are things really going?
First, you will have to plunge into the “wonderful school years,” namely, physics for the 6th grade. Section "Friction forces". We will not dive deeply, ankle-deep. So, let's look at the picture. In front of us is one-eyed Billy Bones driving a Volkswagen. He saw something on the road and slowed down with all his might. From the point of view of physics, both Volkswagen and Billy Bones are all together called a “body”. Forces act on this body. This is the force of gravity that presses the body to the ground mg
, the reaction force of the support
N
, which counteracts it.
In the simplest case, on a horizontal surface, these forces are equal and directed in different directions, and their resultant is zero. In addition to them, another force acts on a moving body - the friction force Ftr
.
The friction force depends on the reaction force of the support and the coefficient of friction; it is directly proportional to them. Or more precisely, it is simply equal to their product: F tr.
= μN .
But the ground reaction force is equal to the mass of the body multiplied by the acceleration of gravity g: N = mg
.
Let's substitute the value of N
into the friction force formula:
F tr.
= μmg Since the gravitational acceleration is the same on the entire planet Earth, we conclude that the friction force depends on the coefficient of friction and the mass of the body, and nothing else.
If some force acts on a thing, it begins to accelerate (remember that from the point of view of physics, braking is also acceleration, only with the opposite sign). According to Newton's second law, this force is equal to mass times acceleration: F = ma
This means that the acceleration is equal to
a = F / m
.
There is only one force acting on our body - the force of friction (the resultant of the others is zero, which means they have no influence). This means a = F tr.
/m , that is, acceleration (deceleration of braking) is equal to the friction force divided by the mass of Billy Bones and his Volkswagen.
But the friction force is equal to F tr.
= μmg .
Let's substitute this value into our formula: a = μmg/m
.
Mass divided by the same mass is reduced. This means a = μg
So, acceleration (in our case, this is the intensity of braking) depends only on the friction coefficient! Whatever the mass of the body, it is reduced, that is, the greater the mass, the greater the friction force will be, and by exactly the same amount.
It seems that everything is already clear. But we need to solve the problem to the end and calculate the braking distance. It's simple. Acceleration a
is equal to the speed
V
divided by the time
t a = V / t
Then
t = V / a = V / μg
According to the Law of Uniformly Accelerated Motion, the distance S
is equal to:
S = at 2 / 2
Then
S = μg (V / μg) 2 / 2 = (V 2 / μg) / 2 = V 2 / 2μg
Driver reaction time and path
Driver reaction time is the time that will pass from the moment the driver detects a danger on the road until the start of taking measures to prevent it.
The driver's reaction path is the distance the vehicle will travel from the moment the driver detects a hazard on the road until the brake pedal is pressed.
Here is the formula for calculating the distance a car will travel when the driver reacts to danger:
(Speed in km/h: 10) x 3 = reaction distance in meters
Calculation example: imagine that you are driving at a speed of 100 km/h along a country road and suddenly an elk runs out onto the road. Calculation: (100 km/h: 10) x 3 = 30 (meters). That is, after you react to a danger on the road, your car will travel about 30 meters. Add to this the braking distance of the car.
Attention: these rules are not scientifically correct formulas and provide only approximate values!
How to improve safety when driving
It is not for nothing that the basic rule of driving states that the driver must keep a sufficient distance from other cars on the road so that there is space for emergency braking and in order not to provoke an accident. But, on the other hand, you should not keep the distance between cars too large. Remember that everything should be in moderation. Here are some driving rules from the experts:
- In city traffic
: Keep a distance of about 15 meters from other vehicles. - On motorways, highways and country roads
: At a speed of approximately 100 km/h, maintain a distance of approximately 50 meters. In poor visibility or on slippery roads, the distance to other cars should be doubled. For example, at a speed of 100 km/h on a slippery road, keep a distance of at least 100 meters from the car in front.
Information publication: Traffic police news, accidents, traffic fines, traffic police, Online traffic rules exam. Technical inspection
A real revelation for novice drivers is the difference in braking distance every time the car moves down the road.
The importance of this parameter is very important; the ability to determine the braking distance of your car will be very useful to the motorist in the future
Formulas for calculating stopping and braking distances, as well as safe distances.
In the theory exam there is a question about the average reaction time of a driver, the correct answer to which is 1 second. The traffic police tickets also contain a question related to safe distance. There are questions regarding braking. But, as they say, a theory is a theory, which, alas, as a rule, has nothing in common with practice.
Firstly, what you taught in the tickets is a theory based on averages and various studies. In fact, the driver's reaction time, stopping and braking distance depend on many factors and cannot be accurately calculated for all cases. Nevertheless, every driver should be able to calculate these parameters at least approximately.
Braking distance of a car at a speed of 60 mph
Body deformation in a collision at a speed of 60 km/h
Stopping distance
also depends not only on the driver, but also on other related factors: the quality of the road, driving speed, weather conditions, the condition of the brake system, the design of the brake system, vehicle tires and many others.
Please note that the weight of the car does not affect the braking distance. This is due to the fact that the weight of the car increases the inertia of the car when braking, thereby preventing braking, but increases the grip of the tires on the road due to the increased weight of the car
These physical properties compensate each other, while having virtually no effect on the braking distance.
The braking speed directly depends on the braking method. Hard brake
all the way, will lead to the car skidding or skidding (if the car is not equipped with an ABS system).
Gradual pressing
The pedal is used when there is good visibility and a calm environment on the road; it is not suitable for emergency situations.
If you press intermittently,
you can lose control, but you can stop quickly.
Stepwise pressing
is also possible (similar in effect to the ABS system).
There are special formulas that allow you to determine the length of the braking distance. We will try to calculate the formula for different conditions, depending on the type of road surface.
Braking distance on dry asphalt
Let's remember physics lessons, where ?
is the coefficient of friction,
g
is the acceleration due to gravity, and
v
is the speed of the car in meters per second.
The situation is as follows: a driver is driving a Lada car whose speed is 60 km/h. Literally 70 meters away is an elderly woman, who, forgetting about safety rules, hastily catches up with a minibus (a standard situation for Russia).
Let's use this very formula: 60 km/h = 16.7 m/sec. Dry asphalt has a friction coefficient of 0.7, g – 9.8 m/s. In fact, depending on the composition of the asphalt, it is from 0.5 to 0.8, but let’s take the average value.
The result obtained from the formula is 20.25 meters. Naturally, this value is appropriate only for ideal conditions, when the car is equipped with high-quality tires and brake pads, the brake system is working properly, and when braking you do not skid or lose control, due to many other idealized factors that do not occur in nature.
Also, to double-check the result, there is another formula for determining the braking distance
:
S = Ke * V * V / (254 * Fs), where Ke is the braking coefficient, for passenger cars it is equal to one; Fs – coefficient of adhesion with coating 0.7 (for asphalt).
We substitute the vehicle speed in km/h.
It turns out that the braking distance is 20 meters for a speed of 60 km/h (for ideal conditions), if the braking is sharp and without skidding.
Braking distance on surface: snow, ice, wet asphalt
BMW cars on test
The friction coefficient helps indicate the stopping distance under different road conditions. Coefficients for different road surfaces
:
- Dry asphalt – 0.7
- Wet asphalt – 0.4
- Rolled snow – 0.2
Let's try to substitute these values into the formulas and find the braking distance values for the road surface at different times of the year and under different weather conditions
:
- Wet asphalt – 35.4 meters
- Rolled snow – 70.8 meters
- Ice – 141.6 meters
It turns out that on ice the braking distance is almost seven times
higher, relative to dry asphalt (as well as the substituted coefficient). The length of the braking distance is affected by the quality of winter tires and physical properties.
Testing has shown that with the ABS system, the stopping distance is significantly reduced, but still, in icy and snowy conditions, ABS does not affect, but rather worsens braking efficiency when compared with a braking system without ABS. However, in ABS, to a large extent, everything depends on the settings and the presence of a brake force distribution system (EBD).
The advantage of ABS in winter
– full control over the control of the car, which minimizes the occurrence of uncontrolled skidding when braking. The principle of operation of ABS is similar to the implementation of stepped braking on cars without ABS.
The ABS system reduces braking distance on: dry and wet asphalt, compacted gravel, road markings.
On ice and compacted snow, the use of ABS increases the braking distance by 15 - 30 meters, but allows you to maintain control over the car, without the car going into a skid. This fact should be taken into account.
What is the braking distance at speeds of 50, 80 and 110 km/h.
As you can see, due to the many variables, it is impossible to create a clear table describing the exact stopping distance of an individual vehicle. This is influenced by both the technical condition of the car and the quality of the road surface.
Average braking distance data for a passenger car with a working system, high-quality tires and normal driver reaction:
| Speed, km/h. | Approximate braking distance, m. |
| 50 | 28 (or six car bodies) |
| 80 | 53 (or 13 car bodies) |
| 110 | 96 (or 24 cases) |
The following conditional situation shows why it is important to stick to the speed limit and not rely on “ideal” brakes. To stop in front of a pedestrian crossing from a speed of 50 km/h to zero, a car will need a distance of almost 30 meters
If the driver violates the speed limit and moves at a speed of 80 km/h, then when reacting at a distance of 30 meters before the crossing, the car will hit a pedestrian. In this case, the speed of the car will be about 60 km/h.
As you can see, you should never rely on the reliability of your car, but it is correct to follow the recommendations, because they are taken from real situations.
How to calculate the braking distance of a car.
Most car owners probably know how quickly a car accelerates. Even if you haven’t measured the acceleration dynamics of your car, you’ve probably looked at the factory specifications of your car, where the automaker usually indicates the minimum possible acceleration time from 0-100 km/h. But now the question is: how long does it take to stop your car? Do you know this? We are sure not. But it turns out that you can calculate the braking distance quite easily using a simple formula. We'll tell you how it's done.
There is no such thing in the Universe or matter that can stop instantly. Likewise, any car, when you press the brake pedal, may not stop immediately. The fact is that in order for a car or any object in our world to stop, it is necessary for it to lose the energy that moves it. As a result, any car has a braking distance that it covers from the moment you press the brake pedal until it comes to a complete stop. This is the braking distance of the car.
But in fact, the braking distance of any car depends not only on its characteristics and braking system, but also on the driver’s reaction when pressing the brake pedal. After all, in order to make a decision about the need to brake and press the brake pedal, time is required, which, although minimal, is enough for the car to cover a long distance. This is especially important at high speeds, where in a few fractions of a second the car travels a considerable distance. So, in the end, in order to calculate the actual braking distance, you need to take into account not only the time and distance traveled by the car from the moment the driver presses the brake pedal until the car stops, but also the time required to make the decision to brake. The fact is that when making a decision to brake, we waste precious seconds. Here's an example:
In addition, even when the brake pedal is pressed, there is still a small loss of time due to the fact that when the brake pedal is pressed, the car, as a rule, does not begin to brake sharply. And in order for the car to actually begin to sharply reduce speed, it is necessary to increase the pressure on the brake pedal (the threshold time required for the required brake pressure in the brake system). Also, all cars have different response times to the brake pedal. Everything here, of course, depends on the design of the brake system and the presence of various electronics that control the car’s brakes.
You won’t believe it, but in order for the car to actually start braking after pressing the brake pedal, almost 1 more second of time is needed. Can you imagine how much this is when driving at high speed? In that extra second you can travel a very long way.